|
 |
 |
 |
 |
 |
 |
(generalized) conchoid |
main |
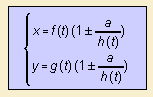with h(t) = √(f2(t)+g2(t))
Given a curve
C1(f(t), g(t)) and a fixed point O(0,0). Draw lines
l through O that intersect C1 in Q. Then the conchoid is defined as the
collection of points P (on l) for which PQ is equal to a constant a.
This conchoid is a generalization of the 1st curve that was named as a conchoid, the (simple) conchoid that is derived from the straight line.
In the following table some conchoids have been collected:
| C1 | O | conchoid of C1 |
| Archimedes' spiral | center | Archimedes' spiral |
| circle | on C1 | limaçon When the constant a is equal to the diameter of C1 the cardioid appears. |
| not on C1 | conchoid of a circle | |
| curve with polar equation r = f(φ) | the origin | curve with polar equation r = f(φ) + a |
| rose | center | botanic curve |
| straight line |
(simple) conchoid |
The conchoid of Dürer does not belong to this family, it is another variation on the conchoid.