spiric section

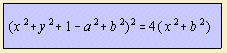
When the plane that intersects the torus is parallel to the axis of the torus, the plane spiric curve turns into the spiric section ¹⁾.
It was the Greek scholar Perseus (150 BC) who investigated the curve, what lead to the name of the spiric of Perseus.

Given a circle of radius 'a' and an axis of revolution in the same plane. The torus or spiric surface can be defined as the surface generated by rotating the circle along a circle of radius r. Then the spiric section is formed by the intersection of the spiric surface with a plane, which is parallel to the axis of revolution.
Let the value of b give the distance of the cutting plane from the center of the torus, and setting r to 1, above formula represents the curve.

For b = 0 the curve consists of two circles.

According to Geminus, Perseus wrote an epigram on his discovery of the curve: "Three curves upon five sections finding, Perseus made offering to the gods...". There have been given different explications of this sentence. Bulmer-Thomas states suggests that Perseus found five sections, but only three of them gave new curves.

When the plane is tangent to the interior of the torus, we get the hippopede.
When b is equal to the inner radius of the torus (c=1 ¹⁾), we get the Cassinian oval.

notes

1) The Cartesian equation can also be written as:

(x²+y²) + 2x² - 2cy² = d

And spiric comes from the Greek word σπειρα for torus.